
Some years ago, too many for my reckoning, I was invited to contribute an article for a special issue of the journal Current Science (Bangalore), on the use of mathematics in different scientific disciplines. I had occasion to read the article again after some 15 years, mainly to cannibalize it for a talk I had to give yesterday, I should confess. Some embarrassment is inevitable on reading something one has written some time ago (I have almost never looked at my Ph D thesis, for example) but I thought that some of it could be shared, so here is an abbreviated essay where I have not removed all the dated bits… The title, of course, acknowledges a great thinker and physicist, Eugene Wigner.
An increasingly quantitative approach within the biological sciences has been accompanied by a greater degree of mathematical sophistication. However, there is a need for new paradigms within which to treat an array of biological phenomena such as life, development, evolution or cognition. Topics such as game theory, chaos theory and complexity studies are now commonly used in biology, if not yet as analytic tools, as frameworks within which some biological processes can be understood. In addition, there have been great advances in unravelling the mechanism of biological processes from the fundamental cellular level upwards that have also required the input of very advanced methods of mathematical analysis. These range from the combinatorics needed in genome sequencing, to the complex transforms needed for image reconstruction in tomography. In this essay, I discuss some of these applications, and also whether there is any framework other than mathematics within which the human mind can comprehend natural phenomena.
It is a commonplace that in recent years the biological sciences have gradually become more quantitative. Far from being the last refuge of the nonmathematical but scientifically inclined, the modern biological sciences require familiarity with a barrage of sophisticated mathematical and statistical techniques.
By now the role of statistics in biology is traditional, and has been historically derived from the need to systematize a large body of variable data. The relation has been two- sided: biological systems have provided a wealth of information for statisticians and have driven the development of many measures, particularly for determining significance, as in the χ2 or Student’s-t tests. Indeed, Galton’s biometrical laboratory was instrumental in collecting and tabulating a plethora of biological measurements, and these and similar data formed the testing ground for a number of statistical theories.
The role of mathematics in biology is more recent. The phenomenal developments in experimental techniques that have helped to make biology more quantitative have necessitated the applications of a number of different mathematical tools. There have been unexpected and frequently serendipitous applications of techniques developed earlier and in a different context. The widespread use of dynamic programming techniques in computational biology, of stochastic context-free grammars in RNA folding, hidden Markov models for biological feature recognition in DNA sequence analysis, or the theory of games for evolutionary studies are some instances of existing methods finding new arenas for their application. There have also been the mathematics and the mathematical techniques that derive inspiration from biology. The logistic mapping, the discrete dynamical system that is so central to chaos theory, arose first in a model of population dynamics. Attempts to model the human mind have led to the burgeoning field of artificial neural networks, while the theory of evolution finds a direct application in the genetic algorithm for optimisation.
Mathematics is about identifying patterns and learning from them. Much of biology is still most easily described as phenomena. The underlying patterns that appear are nebulous, so extracting a set of rules or laws from the huge body of observations has not always been easy. Or always possible since some experiments (like evolution) are unrepeatable, and separating the essential from the inessential can be very difficult. Detail is somewhat more important in the life sciences: often it has been said that the only law in biology is that to every ‘law’, there is an exception. This makes generalizations difficult: biological systems are more like unhappy families. With the exception of natural selection, there are no clearly established universal laws in biology.
This is, of course, in sharp contrast to the more quantitative physical sciences where the unreasonable effectiveness of mathematics has often been commented upon. It might be held that these observations, coming as they do in the twentieth century, comment on a science that has already had about three centuries of development. The earlier stages of the fields that we now call physics or chemistry were also very poorly described by mathematics—there was no general picture beyond a set of apparently unrelated observations, and it required the genius of a Mendeleev, of a Faraday or Maxwell or Einstein to identify the underlying patterns and expose the mathematical structure that lay under some aspects of these fields. This structure made much of the modern physical sciences possible, and led to some of the most accurate verifications of the laws of physics. As predictive theories, relativity and quantum electrodynamics are unparalleled and have achieved astonishing accuracy. In a more complex setting, the seemingly infinite possibilities of organic chemical reactions have found organizational structure in the Woodward–Hoffman rules that combine an elementary quantum mechanics with notions of graph theory to make precise, semiquantitative predictions of the outcome of a large class of chemical reactions. What will it take to similarly systematize biology? Or to rephrase the question, what will the analogous grand theories in biology be?
The inevitable applications of mathematics are those that are a carry-over from the more quantitative physical sciences. As in the other natural sciences, more refined experiments have spearheaded some of these changes. The ability to probe phenomena at finer and finer scales reduces some aspects of biology to chemistry and physics, which makes it necessary to borrow the mathematics that applies there, often without modification. For instance, tomographic techniques rely on a complicated set of mathematical transforms for image reconstruction. These may be largely unknown to the working biologist who uses NMR imaging, but are a crucial component of the methodology, nonetheless. Similarly, the genome revolution was catalysed by the shotgun sequencing strategy which itself relied on sophisticated mathematics and probability theory to ensure that it would work. Several of the problems in computational biology arose (or at least were made more immediate, and their resolution more pressing) by the very rapid increase in experimental power.
The other sort of application of mathematics is, for want of a better descriptor, a systems approach, namely that which is not predicated by the reductionist approach to biology but instead by a need to describe the behaviour of a biological entity in toto.
Even the simplest living organisms appear to be complex, in way that is currently poorly described and poorly understood, and much as one would like, it is not possible to describe in all totality the behaviour of a living organism in the same way as one can the behaviour of, say, a complex material. The promise that there could be mathematical models that capture the essence of this complexity has been held out in the past few decades by several developments, including that of inexpensive computational power which has made possible the study of more realistic models of biological systems. Theoretical developments—cellular automata, chaos theory, neural networks, self-organization— have provided simple mathematical models that seem to capture one or the other aspect of what we understand as ‘complexity’, which itself is an imprecise term. There is one class of applications of mathematical or physical models to biology which attempt to adapt an existing technique to a problem, while another aims to develop the methods that a given problem needs. Each of these approaches have their own value and appeal. In the next sections of this article, I discuss some of the ways in which they have found application in the study of biological systems.

The resonance of the title with those of the well-known essays by Wigner and Hamming is deliberate, as is the dissonance. There are applications in the physical sciences where knowledge of the underlying mathematics can provide very accurate predictions. Comparable situations in the biological sciences may not arise, in part because it may be unnecessary, and in part because biological systems are inherently unpredictable since they are so fundamentally complex. The demands, as it were, that are made of mathematics in the life and physical sciences are very distinct, and therefore, it is very reasonable that the mathematics that finds application in the two areas can also be very different.
Is there any framework other than mathematics within which we can systematize any knowledge? Recent advances in cognitive studies, as well as information that is now coming from the analysis of genomes and genes, suggest that several aspects of human behaviour is instinctual (or ‘hardwired’). That mathematical reasoning is an instinct that we are endowed with is a distinct possibility, and therefore, it may not be given to us (as a species) to comprehend our world in any other manner. This point of view, that it is very natural that we should use mathematics to understand any science, is explored below.
In the last few years there has been a veritable explosion in the study of complex systems. The concept of complexity is itself poorly defined (‘the more complex something is, the more you can talk about it’ ), and as has been pointed out by others, ‘If a concept is not well-defined, it can be abused.’ Nevertheless, there is some unity in what studies of complexity aim to uncover.
A common feature of many complex systems is that they are composed of many interconnected and interacting subunits. Many systems, natural as well as constructed, are, in this sense complex. Examples that are frequently cited apart from those involving living organisms such as ecologies or societies, are the human brain, turbulent flows, market economies or the traffic. A second feature of complex systems is that they are capable of adaptation and organization, and these properties are a consequence of the interconnection and interactions of the subunits. The mathematics of complex systems would thus appear a natural candidate for application to biology. The drawback is that there is, at present, no unifying framework for the study of complex systems although there are some promising leads offered by studies of dynamical systems, cellular automata and random networks.
That the description of phenomena at one level may be inadequate or irrelevant at another has been noted for a long time. Thus the electronic structure of atoms can be understood quite adequately without reference to quarks, and is itself irrelevant, for the most part, when dealing with the thermodynamics of the material of which the atoms are constituents. Schrödinger, in a chapter of his very influential book (Schrödinger, E., What is Life?, Cambridge University Press, Cambridge, 1967) entitled ‘Is Life Based on the Laws of Physics’, observed that with regard to ‘the structure of living matter, that we must be prepared to finding it working in a manner that cannot be reduced to the ordinary laws of physics’. He further contrasted the laws of physics and chemistry, most of which apply in a statistical sense, to biological phenomena, which, even though they involve large numbers of atoms and molecules, nevertheless have nothing of the uncertainty associated with individual properties of the constituent atoms. Indeed, given a radioactive atom, he says, ‘it’s probable lifetime is much less certain than that of a healthy sparrow’.
But even at a given level, it frequently happens that the properties of a system cannot be simply inferred from those of its constituents. The feature of emergence, namely the existence of properties that are characteristic of the entire system but which are not those of the units, is a common feature of systems that are termed complex.
Distinction should be drawn between the complex and the complicated, though this boundary is itself poorly defined. For instance, it is not clear whether or not in order to be deemed complex, a system requires an involved algorithm (or set of instructions). The algorithmic complexity, defined in terms of the length of the (abstract) program that is required is of limited utility in characterizing most systems

Attempts to decode the principles that govern the manner in which new properties emerge—for example the creation of a thought or an idea, from the firing of millions of neurons in the brain, or the cause of a crash in the stock market from the exit poll predictions in distant electoral constituencies—require new approaches. The principles themselves need not necessarily be profound. A simple example of this is provided by a study of flocking behaviour in bird flight. A purely ‘local’ rule: each bird adopts the average direction and speed of all its neighbours within distance R, say, is enough to ensure that an entire group adopts a common velocity and moves in unison. This behaviour depends on the density of birds as well as the size of R relative to the size of a bird in flight. If R is the size of a bird, then each bird flies on its own path, regardless of its neighbours: there is no flock. However, as R increases to a few times that of the bird, depending on the density, there can be a phase transition, an abrupt change from a random state to one of ordered, coherent, flight. And such a system can adapt rapidly: we have all seen flocks navigate effortlessly through cities, avoiding tall buildings, and weaving their way through the urban landscape at high speed.

But there are other aspects of complexity. A (western) orchestra, for instance, consisting as it does of several musicians, requires an elaborate set of rules so that the output is the music that the composer intended: a set of music sheets with the detailed score, a proper setting wherein the orchestra can perform, a specific placement of the different musical instruments, and above all, strict obedience to the conductor who controls what is played and when. To term this a complex system would not surprise anyone, but there is a sense in which such a system is not: it cannot adapt. Should the audience demand another piece of music, or music of another genre, an orchestra which has not prepared for it would be helpless and could not perform. Although the procedure for creating the orchestra is undoubtedly complicated, the result is tuned to a single output (or limited set of outputs). There is, of course, emergence: a single tuba could hardly carry a tune, but in concert, the entire orchestra creates the symphony.
Models like this illustrate some of the features that complex systems studies aim to capture: adaptability, emergence and self-organization, all from a set of elementary rules. The emphasis on elementary is deliberate. Most phenomena we see as complex have no obvious underlying conductor, no watchmaker, blind or not who has implemented this as part of a grand design (Dawkins, R., The Blind Watchmaker, Norton, New York, 1996). Therefore, in the past few decades, considerable effort has gone into understanding ‘simple’ systems that give rise to complex behaviour.
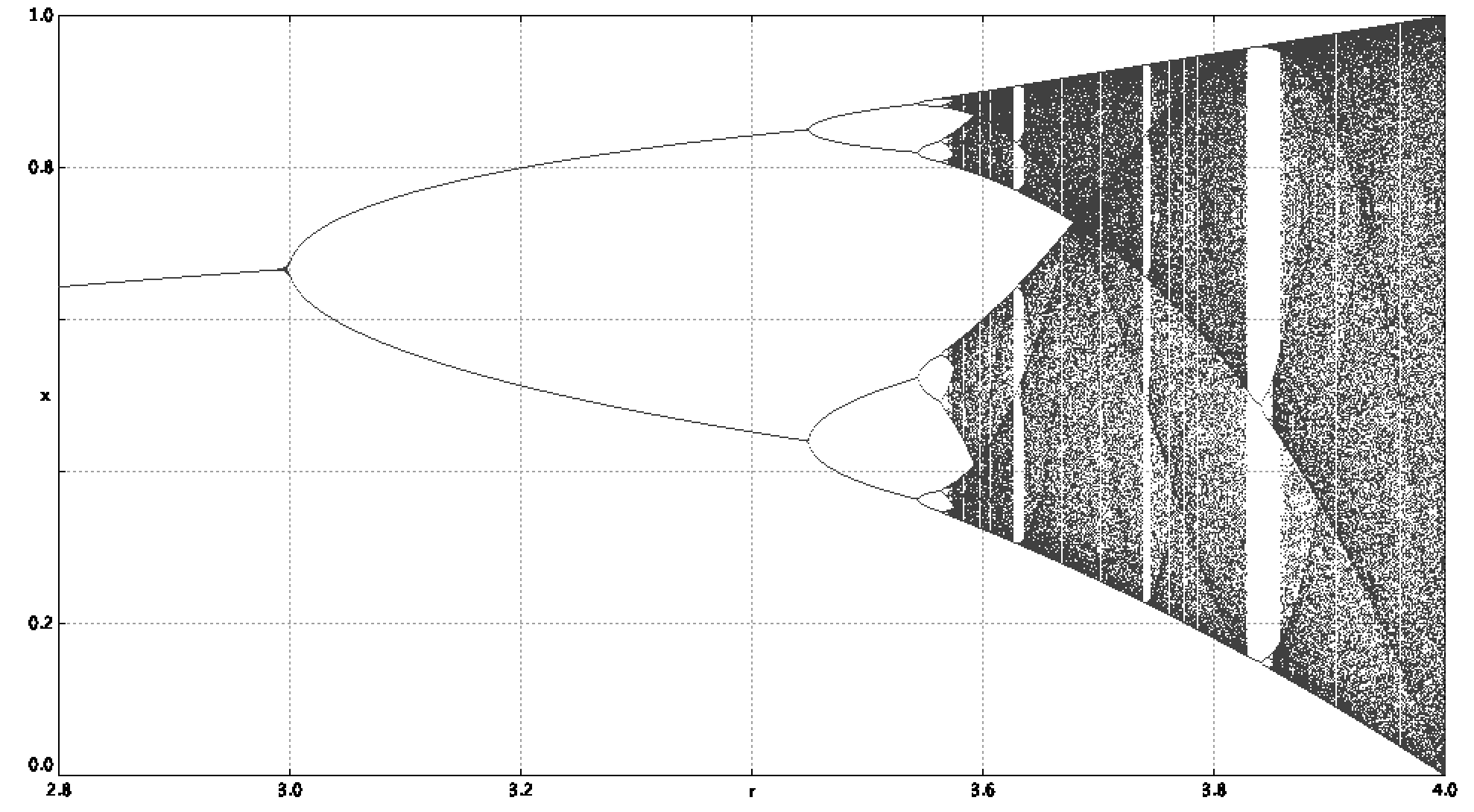
‘Simple mathematical models with very complicated dynamics’, a review article published in 1976 (May, R. M., Simple mathematical models with very complicated dynamics. Nature, 1976, 261, 459) was responsible in great measure for the phenomenal growth in the study of chaotic dynamics. In this article—which remains one of the most accessible introductions to chaos theory— May showed that the simplest nonlinear iterative dynamical systems could have orbits that were as unpredictable as a coin-toss experiment. The thrust of much work in the past few decades has been to establish that complex temporal behaviour can result from simple nonlinear dynamical models. Likewise, complex spatial organization can result from relatively simple sets of local rules. Taken together, this would suggest that it might be possible to obtain relatively simple mathematical models that can capture the complex spatiotemporal behaviour of biological systems.
A number of recent ambitious programs (eCell, A multiple algorithm, multiple timescale simulation software environment, http://www.e-cell.org) intend to study cellular dynamics, metabolism and pathways in totality, entirely in silico. Since the elementary biochemical processes are, by and large, well-understood from a chemical kinetics viewpoint, and in some cases the details of metabolic pathways have also been explored, entire genomes have been sequenced and the genes are known, at least for simple organisms, the attempt is to integrate all this information to have a working computational model of a cell. By including ideas from network theory and chemical kinetics, the global organization of the metabolic pathway in E. coli has been studied computationally. This required the analysis of 739 chemical reactions involving 537 metabolites and was possible for so well-studied an organism, and the model was also able to make predictions that could be experimentally tested. The sheer size of the dynamical system is indicative of the type of complexity that even the simplest biological organisms possess; that it is even possible for us to contemplate and carry out studies of this magnitude is indicative of the analytic tools that we are in a position to deploy to understand this complexity.
In recent years, there has been considerable debate, and an emerging viewpoint, that the human species has an instinct for language. Champions of this school of thought are Chomsky, and most notably, Steven Pinker who has written extensively and accessibly on the issue

The argument is elaborate but compelling. It is difficult to summarize the entire line of reasoning that was presented in The Language Instinct, but one of the key features is that language is not a cultural invention of our species (like democracy, say), but is hard-wired into our genome. Like the elephant’s trunk or the giraffe’s neck, language is a biological adaptation to communicate information and is unique to our species.
Humans are born endowed with the ability for language, and this ability enables us to learn any specific language, or indeed to create one if needed. Starting with the work of Chomsky in the 1950s, linguists and cognitive scientists have done much to understand the universal mental grammar that we all possess. (The use of stochastic context-free grammars in addressing the problem of RNA folding is one instance of the remarkable applicability of mathematics in biology.) At the same time, however, our thought processes are not language dependent: we do not think in English or Tamil or Hindi, but in some separate and distinct language of thought termed ‘mentalese’.
Language facilitates (and greatly enriches) communication between humans. Many other species do have sophisticated communication abilities—dolphins use sonar, bees dance to guide their hive mates to nectar sources, all birds and animals call to alarm and to attract, ants use pheromones to keep their nestmates in line, etc.—and all species need to have some communication between individuals, at least for propagation. However, none of these alternate instances matches anything like the communication provided by human language.
It is not easy to separate nature from nurture, as endless debates have confirmed, but one method for determining whether or not some aspect of human behaviour is innate is to study cultures that are widely spaced geographically, and at different stages of social development. Such cross-cultural studies can help to identify those aspects of our behaviour that are a consequence of environment, and those that are a consequence of heredity. The anthropologist Donald Brown (Brown, D., Human Universals, Temple University Press, Philadelphia, 1991) has attempted to identify human ‘universals’, a set of behavioural traits that are common to all tribes on the planet.
All of us share several traits beyond possessing language. As a species we have innumerable taboos relating to sex. Some of these, like incest avoidance, appear as innate genetic wisdom, but there are other common traits that are more surprising. Every culture, from the Inuit to the Jarawa, indulges in baby talk. And everybody dreams. Every tribe however ‘primitive’, has a sense of metaphor, a sense of time, and a world view. Language is only one (although perhaps the most striking) of human universals. Other universals that appear on the extensive list in his book, and which are more germane to the argument I make below, are conjectural reasoning, ordering as cognitive pattern (continua), logical notions, numerals (counting; at least ‘one’, ‘two’ and ‘many’) and interpolation.
The last few mentioned human universals all relate to a set of essentially mathematical abilities. The basic nature of enumeration, of counting, of having a sense of numbers is central to a sense of mathematics and brings to mind Kronecker’s assertion, ‘God made the integers, all else is the work of man’. The ability to interpolate, to have a sense of a continuum (more on this below), also contribute to a sense of mathematics, and lead to the question: Analogous to language, do humans possess a mathematics instinct?

Dover, New York, 1963.
Writing a century ago, Poincaré had an inkling that this might be the case. ‘… we possess the capacity to construct a physical and mathematical continuum; and this capacity exists in us before any experience because, without it, experience properly speaking would be impossible and would be reduced to brute sensations, unsuitable for any organization;…’ The added emphasis is mine; the observations are from the concluding paragraph of his essay, ‘Why space has three dimensions’.
If mathematics is an instinct, then it could have evolved like any other trait. Indeed, it could have co-evolved with language, and that is an argument that Keith Devlin has made recently (Devlin, K., The Maths Gene, Wiedenfeld and Nicolson, London, 2000).
At some level, mathematics is about finding patterns and generalizing them and about perceiving structures and extending them. Devlin suggests that the ability for mathematics resides in our ability for language. Similar abstractions are necessary in both contexts. The concept of the number three, for example, is unrelated either to the written or spoken word three, or the symbol 3 or even the more suggestive alternate, III. Mathematical thought proceeds in its version of mentalese.
An innate mathematical sense need not translate into universal mathematical sophistication, just as an innate language sense does not translate into universal poetic ability. But the thesis that we have it in the genes begs the question of whether mathematical ability confers evolutionary advantage, namely, is the human race selected by a sense for mathematics?

New York, 1998.
To know the answer to this requires more information and knowledge than we have at present. Our understanding of what constitutes human nature in all its complexity is at the most basic level. The sociobiologist E. O. Wilson has been at the vanguard of a multidisciplinary effort toward consilience, gathering a coherent and holistic view of current knowledge which is not subdivided in subdisciplinary approaches. This may eventually be one of the grand theories in biology, but its resolution is well in the future. We need to learn more about ourselves.
Traditionally, any sense of understanding physical phenomena has been based on having the requisite mathematical substructure, and this tradition traces backward from the present, via Einstein, Maxwell and Newton, to Archimedes and surely beyond.
Such practice has not, in large measure, been the case in biology. The view that I have put forth above ascribes this in part to the stage of development that the discipline finds itself in at this point in time, and in part, to the manner in which biological knowledge integrates mathematical analysis. The complexity of most biological systems, the competing effects that give rise to organization, and the dynamical instabilities that underlie essentially all processes make the system fundamentally unpredictable, all require that the role played by mathematics in the biological sciences is of necessity very different from that in the physical sciences.
Serendipity can only occasionally provide a ready-made solution to an existing problem whereby one or the other already developed mathematical method can find application in biology. Just as, for example, the research of Poincaré in the area of dynamical systems gave birth to topology, the study of complex biological systems may require the creation of new mathematical tools, techniques, and possibly new disciplines.

Our instincts for language and mathematics, consequences of our particular evolutionary history, are unique endowments. While they have greatly facilitated human development, it is also worth considering that there are modes of thought that may be denied to us, as Hamming has observed , similar to our inability to perceive some wavelengths of light or to taste certain flavours. ‘Evolution, so far, may possibly have blocked us from being able to think in some directions; there could be unthinkable thoughts.’ In this sense, it is impossible for us to think non-mathematically, and therefore there is no framework other than mathematics that can confer us with a sense of understanding of any area of inquiry.
In biology, as Dobzhansky’s famous statement goes, nothing makes sense except in the light of evolution. To adapt this aphorism, even in biology nothing can really make sense to us except in the light of mathematics. The required mathematics, though, may not all be uncovered yet.
